2578
Views & Citations1578
Likes & Shares
Aim: To
analyze the clinical factors influencing the human vision corrections and lens
accommodation via the changing of ocular components of human eye in various
applications.
Methods: An
effective eye model is introduced by the ocular components of human eye
including refractive indexes, anterior surface radius of the cornea (r) and
lens (R), the anterior chamber depth(S1) and the vitreous length (S2). Gaussian
optics is used to calculate the change rate of refractive error per unit amount
of ocular components of a human eye (the rate function M).
Results: For
typical corneal and lens power of 42 and 21.9 diopters, the rate function Mj
(j=1 to 4) are calculated for a 1% change of r and R: M1=+0.485, M2=-0.063, and
1.0 mm change of S1 and S2: M3=+1.35, M4=-2.7 diopters/mm. These rate functions
are used to analyze the clinical outcomes in various applications including
laser in situ keratomileusis (LASIK) surgery, corneal cross linking (CXL)
procedure, femtosecond laser surgery and scleral ablation for accommodation.
Conclusion: Using
Gaussian optics, analytic formulas are presented for the change of refractive
power due to various ocular parameter changes. These formulas provide useful
clinical guidance in refractive surgery and other related procedures.
INTRODUCTION
The IBM
patent (1983) of UV laser for organic tissue ablation was developed into
clinical application for the first human trial of (photorefractive keratoplasty
(PRK) in 1987 and followed by US FDA approval in 1995. The flying-spot scanning
technology invented by Lin (US 1991 patent) leading to the customized LASIK
which was US FDA approved in 2002. The combined technologies of scanning laser,
eye tracking, topography and wavefront sensor advance the corneal reshaping
(the refractive surgery) one step further from the conventional ablation of
spherical surface to the customized ablation of aspherical surface. Therefore,
the theory (or mathematics) behind LASIK is also expanded from the simple
paraxial formula to the high-order nonlinear formulas involving the change of
the corneal asphericity and the LASIK-induced surface aberrations. Most of the
existing LASIK monograms are based on spherical corneal surface. The customized
nomograms require aspherical surface in order to minimize the optical
aberrations [1-4].
Besides the 193 nm excimer laser, various laser systems/procedures were
developed during 1995-2000, including [1]: laser thermal keratoplasty
(LTK, using Ho: YAG), diode laser TK
(using diode laser at 1540 nm), radio frequency and conducting keratoplasty (RF
and CK) designed for hyperopia corrections; UV solid state lasers (213 nm for
PRK), YAG pico-second-PRK, Mini-Excimer for PRK etc. Technologies developed in
the 2000’s include: eye-tracking device (Lai, Nvatek), microkeratome, Elevation
map, topography-guided LASIK, wavefront for customized LASIK (Tracey),
presbyopia treatment using SEB (Schachar) and laser scleral-ablation for
presbyopia (Lin); accommodative IOL. More recently, femto-second lasers are
developed for flat cutting, stroma ablation and cataract. UV-light and
riboflavin activated corneal cross linking (CXL) has been developed for
clinical use for various corneal deceases such as corneal keratoconus, corneal
keratitis, corneal ectasia, corneal ulcers, and thin corneas prior to LASIK vision
corrections.
Combined technology of CXL-PRK, CXL-intra stroma-femto-laser pocket,
CXL-phakic-IOL, CXL-IC-ring. Summary of various ophthalmic lasers is shown in Table 1. Summary of lasers for vision
corrections are shown in Figure 1 and 2,
cataloged by the treatment arears of corneal surface (6-8 mm), scleral (8-13
mm), intrastroma, lens and retina.
MATERIALS AND
METHODS
We will present various applications and the related theoretical
background (or mathematical formulas) including: laser in situ keratomileusis (LASIK) surgery,
femtosecond laser surgery, corneal cross linking (CXL) and accommodative IOL.
Human ocular optics
As shown in Figure 3, an
effective eye model was developed for comprehensive description of human ocular
optics based on Gaussian paraxial approximation [4]. The refractive error (De)
is given by
De = 1000 [n1/(L-L2) - n1/ F]
(1)
where n1 is the refractive index of the aqueous humor, L is the axial
length, L2 is position of the system second principal plane and F is the system
effective focal length (EFL). The system total power is given by D=1000n1/F (D
in diopter, F in mm) which is determined by the corneal (D1) and lens power
(D2) as follows: D = D1 + D2 – S(D1D2)/(1000n1), with corneal power D1 = 1000
[(n3-1)/r1 – (n3-n1)/r2] + bt; and lens power given by D2 = 1000 [(n4-n1)/R1 +
(n4-n2)/R2] –aT;
wherenj (j=1, 2, 3, 4) are the refractive index for the aqueous,
vitreous, cornea and lens, respectively. The anterior and posterior radius of
curvatures (in the unit of mm) of the cornea and lens are given by (r1, r2) and
(R1, R2), respectively, where the only concave surface R2 is taken as its
absolute value in this study. Finally, S is the effective anterior chamber
depth, related to the anterior chamber depth (ACD), S1, by S=S1+P11+0.05 ( in
mm), where P11 is the distance between the lens anterior surface and its first principal
plane, and 0.05 mm is a correction amount to include the effect of corneal
thickness (assumed to be 0.55 mm) [2,3]. The thickness terms in Eq.(2.b) and
(2.c) are given by b=11.3/(r1r2), a=4.97/(R1R2) for refractive indexes of n1 =
n2 = 1.336, n3 = 1.377 and n4 = 1.42; and t and T are the thickness of the
cornea and lens, respectively.
As shown in Fig. 1, using L-L2=X+ SF/f, with X=L-S-aT+0.05, and aT and
0.05 are the correction factors for the lens and cornea thickness, Eq.(1) may
be rewritten in an effective eye model equation [4]
De = Z2 [1336/X – D1/Z – D2] (2)
where
Z=1-S/f , with f (in mm) is the EFL of the cornea given by f=1336/D1, and the
nonlinear term k is about 0.003 calculated from the second-order approximation
of SF/(1336f). The nonlinear term may also be derived from the IOL power
formula [5]. We note that in Eq. (3), X, Z, S and f are in the unit of mm; D1,
D2 and De are in the unit of diopter; and the 1336 is from 1000x1.366 in our
converted units.
The Rate functions
To find the change of refractive error (De) due to the change of ocular
components, the anterior chamber depth (S1) and vitreous length (S2), related
to the axial length by L=S1+S2+T. The derivative of the refractive error (De)
with respect to these ocular parameter change (Qj) given by Mj=dDe/dQj, defines
the rate function, or the change of De per unit amount change of Qj. The rate
function for Qj is anterior curvature of cornea (r), lens (R), S1 and S2,
defined by M1=dDe/dQj were previously derived and given by [4].
M1 = +378/r2
(3.a)
M2= +82.75 (Z/R)2, (3.b)
M3= 1336 (1/F2 – 1/f2) (3.c)
M4= - 1336/F2
(3.d)
where f and F (both in mm) are the corneal and
system EFL given by f=1336/D1 and F=1336/D; and Z=1-S/f. Eq. (3.c) is for the
rate function for the lens anterior curvature (R) change in femtosecond
procedure to be discussed later. For typical values of r=7.8 mm, R=10.2 mm,
S=6.0, S1=3.5 and S2=16.0 mm, axial length of L=3.5 + 16 + 4 = 23.5 mm, the
corneal and lens power are calculated D1=42 diopter, D2=21.9 diopter and total
power, from Eq.(2.a), D=D1+0.811D2=59.8 diopter, The typical rate functions are calculated for
a 1% change of r,R, S1, S2 ( in
diopters): M1=+0.49, M2=+0.053,
M3=+1.35, and M4=-2.67 diopter/mm. Clinical applications of above rate
functions are discussed as follows.
RESULTS AND
DISCUSSIONS
We will present various applications related to the formulas presented
in this paper, including: laser in situ keratomileusis (LASIK) surgery, corneal
cross linking (CXL) procedure, femtosecond laser surgery and accommodative IOL.
Greater details are described as follows.
LASIK procedure
A procedure called laser in situ keratomileusis (LASIK), where one
diopter correction only requires an ablation depth about 8 to 11 microns of the
corneal central thicknessor a corresponding change of r1 about 0.16 mm. In
LASIK procedure, the refractive power change is defined by the difference of
the preoperative (R) and postoperative (R') front surface radius of the cornea,
given by D = 377(1/R – 1/R'), where D in
diopter (or 1/m) and R and R' in mm. Therefore, myopia (D<0), R'>R and
hyperipia (D>0), R'
The central ablation depth for a 3-zone myopic correction is given by
[3,5]
H’(3-zone) = RxH(single-zone)
(4.a)
H(single-zone) = (DW2/3)(1+C) (4.b)
Where, W is the diameter of the outer ablation zone having a typical
value of 6.5 to 7.5 mm; C is a nonlinear correction term given by C= 0.19
(W/r1)2, r is the corneal anterior radius of curvature. For
examples, for r1=7.8 mm, (or a K-reading of K=337.R1=43.2 D), C = (11.2,
13.2,16.5) % for W =(6.0, 6.5, 7.0) mm. The reduction factor R=(0.70 to 0.85)
depending on the algorithms used. For example, comparing to a single zone with
W=6.5 mm, a 3-zone depth will reduces to 71.6% (or R = 0.716) when an inner
zone 5.5 mm and an outer zone 6.5 mm are used. Furthermore, in a LASIK system,
the input pre-operative parameter of the treated eye must include the K values
which affect the laser ablation depth via the nonlinear term of Eq. (4.b).
Modern customized LASK parameters includes: D, K, and Q-value to correct the
asphericity and the LASIK-induced surface aberrations [3,5].
Age dependent lens
power
It was reported that the change in the refractive index gradient of the
lens cortex has a substantial factor in the contribution to the onset and
progress of presbyopia [6], where an age-dependent equation for an equivalent
lens index neff=1.441 – 0.00039 x Age (in year) was proposed to explain the
lens paradox [7]. Lens index decreases from 1.434 to 1.416 (about 1.25%
decrease) between 20 and 65 years of age to compensate the more convex shape of
aged-lens, given by R1=12.9 – 0.057xAge and R2=6.2-0.012 x Age [7], which would
have caused a myopia rather than presbyopia, if neff would not be
age-dependent. Above statements have been known, but only qualitatively. The
formula shows that a hyperopia shift of 2.47 x 1.25% = 3.1 diopter is
associated to this proposed index decrease of 1.2%. The commonly accepted
estimation of dDe due to the change of lens index was based on a conversion
factor (CF=Z2) of 80% which ignored the contribution from the second
principal plane, in comparing to our new value of CF=(65% to 75%).
Accommodative IOL
(AIOL)
For
patient after cataract, an AIOL in an aphakic eye may be implanted for vision
correction to see both near and far. The accommodation formulas for M1 and M2
can be used to calculate the accommodation amplitude of the AIOL. Our
calculations show the typical values of M3=+1.35, and M4=-2.67 diopter/mm.
These formulas can also be used to calculate the power error of the piggy-back
IOL due to mis-position [8]. Figure 4shows
the dual optic for AIOL showing 2-component model for lens accommodation.
Femtosecond laser surgery
One may
use a femtosecond laser (so called SMILE) to ablate or remove a small portion
of the lens and change its curvature (R), where each 1% reduction may cause a
0.05 to 0.06 diopter change, based on our formula for M2, see Eq. (3.b). This
procedure is not as effective as that of corneal ablation (LASIK) given by M1
in Eq. (3.a). Therefore, one may ablate the lens to restore a 40% change of R
resulting 2.0 to 2.4 diopter accommodation. The current femtosecond laser has a
very low average power and therefore lens ablation could take a much longer
time than a corneal surface ablation in LASIK.
Scleral ablation for presbyopia treatment
Scleral
laser ablation (using Er: YAG laser at 2.94 um) and band expansion have been
used to increase the space of the ciliary-body and zonus such that
accommodation is improved by two components [9,10]: the lens translation and
the lens shaping which are given by, respectively, M3 and M2. For older and/or
harder lens, the accommodation is mainly attributed by the lens translation (or
S1 change), whereas lens shaping dominates the power change in young or soft
lens. It was known that change of the rear surface of the lens is about
one-third of the front surface during accommodation [9,10].
Scleral ablation for glaucoma treatment
As shown
by Figure 5
besides presbyopia treatment, Er: YAG laser can also be used for glaucoma
treatment, followed by a collagen matrix refilling to stable the outcomes.
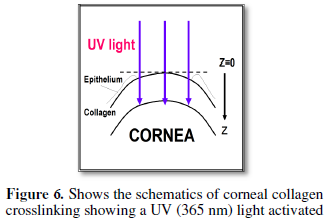
Cornea cross linking
Depending
on the ocular location of the corneal cross linking (CXL) procedure, the new
applications of CXL include examples shown as follows [11,13]:
(1) For CXL applied inside the corneal stroma,
correction of low myopia is possible and may be measured by the K-value
deduction after CXL; where 2% reduction of K-value may cause a 0.9 to 1.1
diopter myopic correction, based on the formula for M1, see Eq. (3.a), with
K=337/r.
(2) For
CXL applied to the orbital scleral tissue, one may stop or reduce the abnormal
axial length (L) growth rate in high myopic eyes; where each 1.0 mm increases
of L may cause 2.2 to 2.8 diopter change, based on our formula for M4, see Eq.
(3.d), assuming that the axial grow is dominated by S2.
(3) For
CXL applied to the corneal stroma postoperatively for procedures such as
conduction keratoplasty (CK), diode laser thermal keratoplasty (DTK), the
postoperative regression due to unstable thermal shrinkage may be stabilized by
CXL process. Eq. (3.a) for M1 may be used to estimate the amount of
postoperative regression reduced by CXL.
(3)
Combined CXL with OK-lens or RPG-lens to stabilize regress. The reshaping
effect of corneal anterial surface may be estimated by M1 of Eq. (3.a).
CONCLUSION
Using Gaussian optics, we have presented analytic formulas for the change of refractive power due to various ocular parameter changes. These formulas provide useful clinical guidance in various applications including LASIK surgery, corneal cross linking (CXL) procedure, femtosecond laser surgery and scleral ablation for accommodation. Accuracy of our formulas for human eyes would depend on individual ocular parameters, which were taken as their averaged values in our calculations.
1.
Lin
JT Progress of the 30-year Laser Vision Technology, J Ophthal Research and
Clinics.
2.
Lin
JT (1994) Mini-excimer laser corneal reshaping using a scanning device. Proc
SPIE 2131: 228-236.
3.
Lin
JT (1995) Critical review on refractive surgical lasers. Opt. Engineer 34:
668-675.
4.
Lin
JT (2016) Gaussian Optics Analysis for Human Eyes with Application for Vision
Corrections. Ophthalmol Res 6: 1-5.
5.
Lin
JT (2005) A new formula for ablation depth in 3-zone LASIK. J Refract Surg 21:
413-414.
6.
Rosem
AM, Denham DB, Fernandez V et al. (2006) In vitro dimensions and curvatures of
human lenses. Vision Res 46: 1006-1009.
7.
Garner
LP, Yap MKH (1992) Change in ocular dimensions and refraction with
accommodation. OphthalPhysiol Opt 17: 12-17.
8.
Lin
JT (2005) New formulas comparing accommodation in human lens and intraocular
lens. J Refract Surg 21: 200-201.
9.
Lin
JT, Mallo O (2003) Treatment of presbyopia by infrared laser radial
sclerectomy. J. Refract Surg 19:465-467.
10.
Lin
JT, Jiang MS, Hong YL, et al. (2010) Analysis and applications of accommodative
lenses for vision corrections. J Biomed
Optics 16.
11.
Lin
JT. Photochemical Kinetic modeling for oxygen-enhanced UV-light-activated
corneal collagen crosslinking. Ophthalmology Research, 2017;7:1-8. DOI:
10.9734/or/2017/35032.
12.
Lin
JT, Cheng DC (2017) Modeling the efficacy profiles of UV-light activated
corneal collagen crosslinking. PloS One 12:e0175002.
13.
Sun
M, Zhnag F, Ouyang B, et al. (2018) Study of retina and choroid biological
parameters of rhesus monkeys eyes on scleral collagen cross-linking by
riboflavin and ultraviolet A. PlosOne.
QUICK LINKS
- SUBMIT MANUSCRIPT
- RECOMMEND THE JOURNAL
-
SUBSCRIBE FOR ALERTS
RELATED JOURNALS
- Journal of Cardiology and Diagnostics Research (ISSN:2639-4634)
- Stem Cell Research and Therapeutics (ISSN:2474-4646)
- Journal of Forensic Research and Criminal Investigation (ISSN: 2640-0846)
- International Journal of Clinical Case Studies and Reports (ISSN:2641-5771)
- Journal of Cell Signaling & Damage-Associated Molecular Patterns
- International Journal of AIDS (ISSN: 2644-3023)
- Journal of Alcoholism Clinical Research